Course Lines are Great Circles

The problem in creating a chart projection lies in developing a method for transferring the

meridians and parallels to a developable surface that will preserve certain desired

characteristics.

Constant Scale: If the chart scale is, for example, "one inch equals one hundred miles,"

then it is desirable that the scale be constant and accurate in every direction for the entire

area covered by the chart.

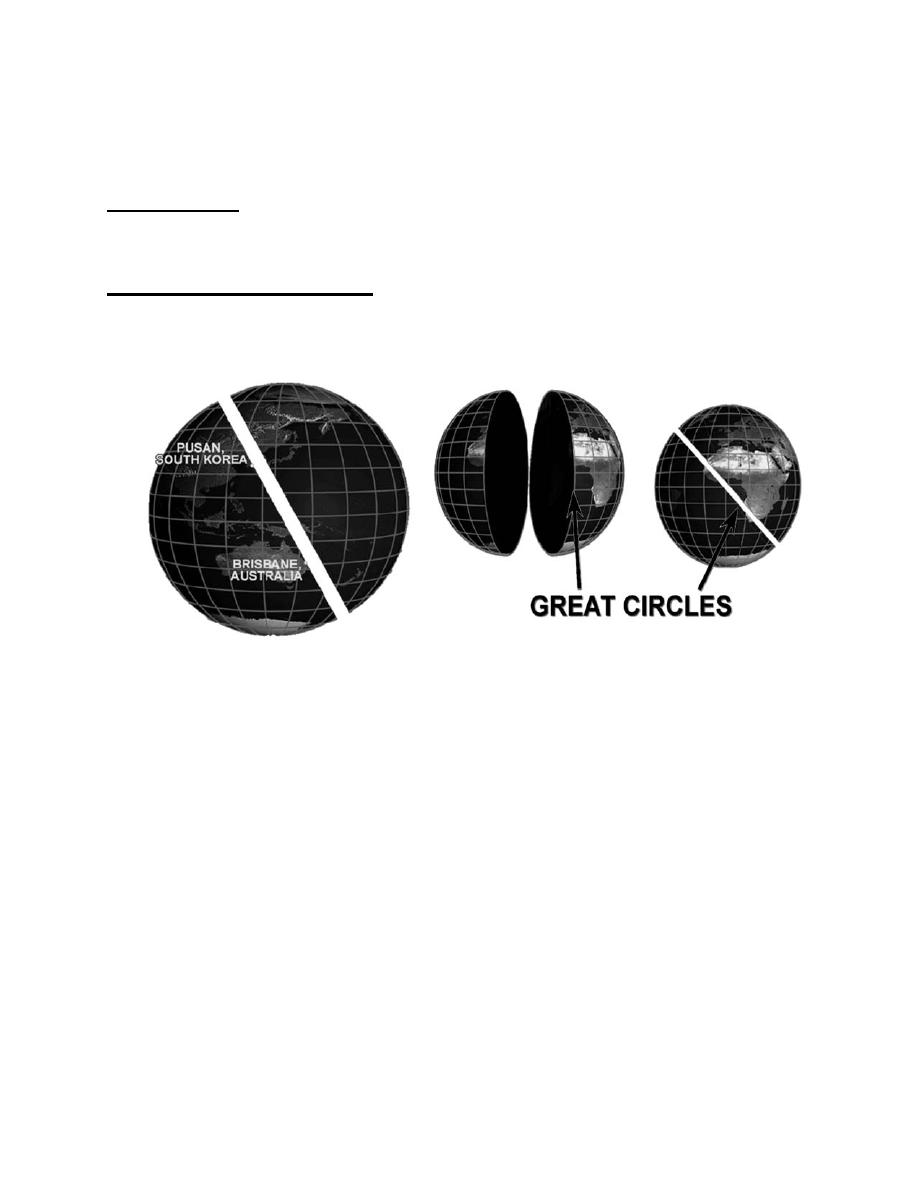
Course Lines are Great Circles: A great circle is a circle formed by continuing the arc

inscribed by connecting the shortest distance between two points on a sphere. Further

defined, it is a circle whose plane passes through the earth's center, dividing the earth into

two equal halves. Several great circles are shown in Figure 4.2-2.

Figure 4.2-2 Great Circles

Notice that great circles are not limited to being horizontal or vertical. They can be at any

angle that divides the sphere into two equal halves. The reason great circles are important

is that a great circle route is the shortest distance between two points, saving both time and

fuel. Only one parallel, the equator, forms a great circle. However, all meridians are great

circles since they vertically bisect the earth.

Simply stated, the intersection of a sphere and a plane is a circle - a great circle if the

plane passes through the center of the sphere and a small circle if it does not.

LAMBERT CONFORMAL PROJECTION

The most widely used projection is the Lambert Conformal Projection. It is referred to as a

"conic" projection because it is developed by placing a secant cone over the earth,

intersecting the earth at two lines of latitude called "standard parallels." The development

of a Lambert Conformal chart projection is illustrated in Figure 4.2-3.

4.6-22