Air Combat Maneuvering
Background
Then we will discuss how you can successfully manage your energy based on concepts related to
operational maneuverability.
THE EGG
Figure 1 depicts “the egg,” representing a three-dimensional sphere showing the effects of gravity as you
maneuver in all planes. You already know, or at least have been introduced to, all the concepts and
principles we are about to discuss. However, outflying the bogey depends on what you know about your
airplane, the arena, and the bogey. Let’s first take a look at turn performance.
Horizontal Maneuvering
The most basic of all aerodynamic principles states that an aircraft, to maintain straight-and-level flight,
must generate exactly 1 g to overcome the effects of gravity. Because the amount of lift required to
maintain 1-g flight is based on the weight of the aircraft (excluding the effects of drag), the vector
representing gravity remains constant as long as the weight of the aircraft remains constant.
RGb
RGa
EFFECTIVE
EL
LIFT FOR
EL
LFb
BOTH PLANES
LFa
80 AOB
60 AOB
G
G
GRAVITY
LF = LOAD FACTOR
G = GRAVITY
RG = RADIAL G
Aircraft a
Aircraft b
EL = EFFECTIVE LIFT
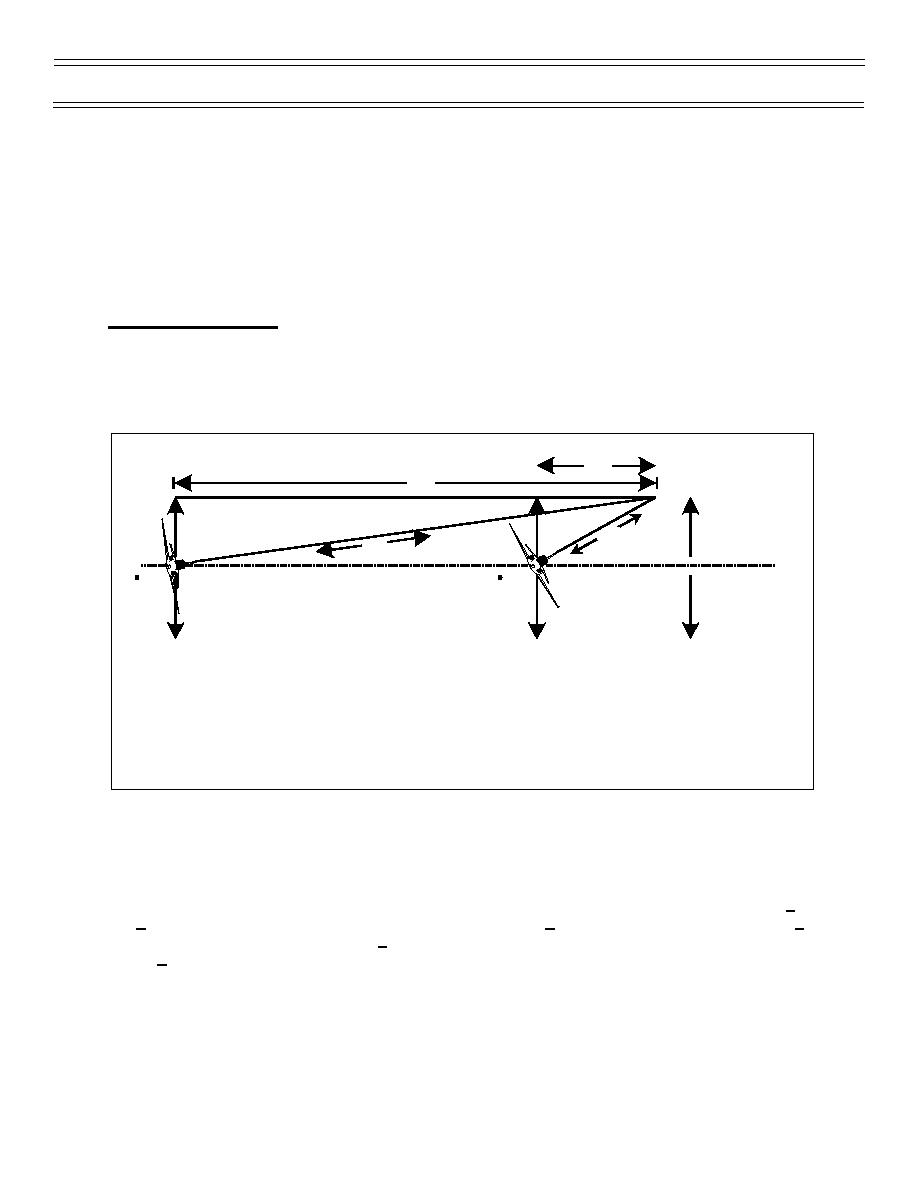
Figure 2: HORIZONTAL MANEUVERING
An aircraft in a turn at any angle of bank (AOB) must generate additional load factor in order to meet the
same effective lift. The load factor increases because your lift vector is moved out of the pure vertical. If
we assume that the effective lift of the aircraft opposes gravity (which is a constant force), the load factor
will vary according to how tightly you want to turn the aircraft. As you can see in Figure 2 , both aircraft a
and b are in level turns at a constant true airspeed (TAS). Aircraft a is in an 80-degree AOB and aircraft b
is in a 60-degree AOB. Because aircraft a is turning at an 80-degree AOB, his load factor is greater than
aircraft b turning at a 60-degree AOB. Notice that because gravity and the effective lift remain constant
forces, the resultant vector, referred to as “radial g,” actually turns the aircraft. Radial g is the horizontal
component of lift. If you pull harder in a turn, which is indicated on your accelerometer and referred to as
“indicated g,” you are increasing the load factor. Depending on your situation (your snapshot in time), this
triangle will change. Simply put, the larger the radial-g vector, the better the turn performance. As you see
in Figure 2, in a purely horizontal turn, the greater the AOB, the greater the load factor to maintain effective
lift. This greater load factor produces greater induced drag, resulting in a higher energy loss. As you will
see, you will want to avoid the pure horizontal because of this.
T-45C Revision 1
Page 4


 Previous Page
Previous Page
